|
음수와 관련된 몇 가지 자료를 링크하겠습니다 <음수의 역사> 음수를 크기에 해당하는 양 http://news.khan.co.kr/kh_news/khan_art_view.html?art_id=200707311147102 <왜 음수 X 음수는 양수인가? > 음수끼리의 곱이 왜 양수가 되는지에 대한 설명 블로그입니다 http://cafe.daum.net/TCP62/3rxO/401?q=%EC%9D%8C%EC%88%98%EC%9D%98%20%EC%9D%B4%ED%95%B4 |
Start a blog to organize and share what you have studied alone. I mainly do theory of relativity and quantum mechanics, and also think about physics stories that elementary and junior high and high school students can understand.
2020년 7월 30일 목요일
동서양 고대 수학(2) - 동양 (구장산술)
2020년 7월 29일 수요일
동서양 고대 수학(1) - 서양(린드 파피루스)
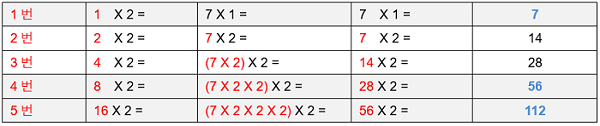
- 1단계) 첫 번째 수인 25에 대해서 2의 거듭제곱 중 25를 넘지 않는 가장 큰 수를 찾는다(16).
- 2단계) 25에서 1단계에서 찾은 16을 빼면, 9가 남는데 1단계에서 처럼 9를 넘지 않는 가장 큰 2의 거듭제곱을 찾는다(8).
- 3단계) 2의 거듭제곱으로 찾아지지 않아, 종료되고 1이 남는다.
- 따라서, 25 = 16+8+1로 분해된다.
25 X 7 = (16+8+1) X 7=16 X 7 + 8 X 7 + 1 X 7 = 112 + 56 + 7
- 1단계) 빵 1개를 3등분합니다. 그러니, 총 조각은 27(=9X3)가 되겠죠. 27개 조각 중 20개는 각자에게 2개씩 10명에게 줍니다. 그럼, 남아 있는 조각은 7개입니다. 7개는 빵 1개를 3등분한 조각이니까. 빵 2개와 1/3 조각이 되는 거죠.
- 2단계) 남아 있는 7개 조각 중 6개 조각은 다시 빵 2개가 됩니다. 남은 빵 2개를 각각 5등분하여 10개 조각을 만들어서 10명에게 1/5 조각 씩 주면 남은 빵은 1/3조각만 남게 됩니다.
- 3단계) 1/3 조각을 30등분하고 각자 3조각씩 나누면 10명에게 공평하게 나눠 지게 됩니다.
2020년 7월 27일 월요일
(Shankar) 5.4. The Single-Step Potential: A Problem in Scattering (2)
- This Case is somewhat different from the case of free particles. Therefore, it would be better to see it compared to the procedure in the Free Particle.
- Golwala's Notation is difficult to match exactly with Shankar's Notation, so I quoted Golwala's Notation as it is and refer to the table compared to Shankar's Notation.
- The part that describes my personal commentary is marked as "My Commentary", so just for reference.
- The part that I do not understand personally was marked as "I don’t understand ???".
- The pictures in Shankar's book are omitted, see Shankar Book
5.4.2. finding the fate of the incident wave packet
■ Shankar’ Book
○
Consider the step potential
(Fig. 5.3)
\(V\left( x \right)\left\{ {\begin{array}{*{20}{c}}{ = 0\;\;\;\;\;\;\;\;x < 0\;\;\;\left( {region\;\;I} \right)}\\{ = {V_0}\;\;\;\;\;x > 0\;\;\;\left( {region\;\;II} \right)}\end{array}} \right.\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\left( {5.4.1} \right)\)
Such an abrupt change in potential is
rather unrealistic but mathematically convenient. A more realistic transition
is shown by dotted lines in the figure.
Imagine now that a classical particle of
energy \(E\) is shot in from the left (region I) toward the step. One expects
that if \({\rm{E}} >
{V_0}\), the particle would climb the barrier
and travel on to region II, while if \({\rm{E}} < {V_0}\) , it would
get reflected. We now compare this classical situation with its quantum
counterpart.
○
First of all, we must consider an initial
state that is compatible with quantum principles. We replace the incident particle
possessing a well-defined trajectory with a wave packet
Though the detailed wave function will be
seen to be irrelevant in the limit we will consider, we start with a Gaussian,
which is easy to handle analytically:
This packet has a mean momentum \({p_0} = \hbar {k_0}\), a mean position \(X = - a\) (which we take to be far away from the step), with uncertainties
We shall be interested in the case of large
\(\Delta \), where the particle has essentially welldefined momentum \(\hbar {k_0}\) and energy \({E_0}
\simeq {\hbar ^2}k_0^2/2m\). We first consider
the case \({E_0} >
{V_0}\).
After a time \(t \simeq a{\left[ {{p_0}/m}
\right]^{ - 1}}\), the packet will hit the step
and in general break into two packets: \({\psi _R}\), the reflected packet,
and \({\psi _T}\), the transmitted packet (Fig. 5.3). The area under \({\left| {{\psi _R}} \right|^2}\) at large \(t\) is the probability of finding the particle in region I in the
distant future, that is to say, the probability of reflection. Likewise the
area under \({\left|
{{\psi _T}} \right|^2}\) at large \(t\)
is the probability of transmission. Our problem is to calculate the reflection
coefficient
■ finding the fate of the incident wave packet, \({\psi _I}\)
○
(Step1, Shankar) Solve for the normalized eigenfunction of the step potential Hamiltonian, \({\psi _E}\left( x \right)\)
In region I, as \(V = 0\), the (unnormalized) solution is the familiar one:
In region II (see Eq. (5.2.2))
Of interest to us are eigenfunctions with \(D = 0\), since we want only a transmitted (right-going) wave in region II, and incident plus reflected waves in region I.
♦ (My commentary) In region II, In Region II, there is no left-going wave, so D=0. Region I has both Incident\(\left( {A{e^{i{k_1}x}}} \right)\) and reflected\(\left( {B{e^{ - i{k_1}x}}} \right)\) waves, so \(A \ne 0\) and \(B \ne 0\).
If we now impose the continuity of \({\psi _E}\left( x \right)\) and its derivative at \(x = 0\).
\(A + B = C\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\left( {5.4.7} \right)\)
\(i{k_1}\left( {A - B} \right) = i{k_2}C\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\left( {5.4.8} \right)\)
\(B = \left( {\frac{{{k_1} - {k_2}}}{{{k_1} + {k_2}}}} \right)A = \left( {\frac{{{E^{1/2}} - {{\left( {E - {V_0}} \right)}^{1/2}}}}{{{E^{1/2}} + {{\left( {E - {V_0}} \right)}^{1/2}}}}} \right)A\;\;\;\;\;\;\;\left( {5.4.9} \right)\).
\(C = \left( {\frac{{2{k_1}}}{{{k_1} + {k_2}}}} \right)AA\;\;\;\;\;\;\;\left( {5.4.10} \right)\)
\({\psi _E}\left( x \right) = A\left[ {\left( {{e^{i{k_1}x}} + \frac{B}{A}{e^{ - i{k_1}x}}} \right)\theta \left( { - x} \right) + \frac{C}{A}{e^{i{k_2}x}}\theta \left( x \right)} \right]\;\;\;\;\left( {5.4.11} \right)\)
where
\(\theta \left( x \right) = \left\{ {\begin{array}{*{20}{c}}{1\;\;\;\;\;\;if\;x > 0}\\{0\;\;\;\;\;\;if\;x < 0}\end{array}} \right.\)
Since to each \(E\) there is a unique \({k_1} = + {\left( {2mE} \right)^{1/2}}\), we can label the eigenstates by \({k_1}\), instead of \(E\). Eliminating \({k_2}\) in favor of \({k_1}\) , =
\({\psi _E}\left( x \right) = {\psi _{{k_1}}}\left( x \right) = A\left[ {\left( {{e^{i{k_1}x}} + \frac{B}{A}{e^{ - i{k_1}x}}} \right)\theta \left( { - x} \right) + \frac{C}{A}{e^{i\sqrt {k_1^2 - \frac{{2m}}{{{\hbar ^2}}}{V_0}} \;x}}\theta \left( x \right)} \right]\;\;\;\;\;\left( {5.4.12} \right)\)
○(Step2, Golwala) propagator \(\left( {U\left( t \right)}\right)\)
- Although not mentioned in the shankar book,
it is detailed in the Golwala Lecture Note. Therefore, we summarize the
contents of the Golwala Lecture Note.
where is the state whose position representation has wavenumber \({k_1} = k\) in region I. By analogy
to the free particle case, we integrate over \(k\)
instead of \(E\) to count states correctly and have the correct differential in the
integral.
Negative \(k\)
corresponds to states that are initially left-going (\(A = 0\) and \(c \ne
0\))
the \(\theta \) function is necessary
because there are no such states for \(0 < E < {V_0}\) for \({V_0} > 0\).
(I don’t understand ???)
♦ (My commentary) Since only right-going is considered, the second term in \(U\left( t \right)\) can be ignored.
Therefore, in Region I, the propagator
becomes the same as that of the Free Particle.
○(Step3, Golwala) Can we do the integral
in closed form as we did for the free particle? No!
The -basis matrix elements of \(U\left( t \right)\) are given by taking the product with on the left and on the right, giving
(I don’t understand \(\theta \left( { - {k_V} - k}
\right)\). ???)
Can we do the integral in closed form as we
did for the free particle? Even for \(x \le 0\) and \(x' \le 0\), where the wavefunctions are free-particle-like, the integral cannot
be done in the same way because of the absence of left-going states for \(0 < {E_k} < {V_0}\) For \(x
> 0\) or \(x' > 0\) the wavefunctions are either decaying exponentials or have an
argument \({k_2}\) that is not related to in the usual simple way, so the integral certainly cannot be done in
the same way for them.
○ (Step4, Shankar) Calculate the initial wave function \({\psi_I}\left( {x,0} \right)\)
First of all, we must consider an initial
state that is compatible with quantum principles. We replace the incident
particle possessing a well-defined trajectory with a wave packet
Though the detailed wave function will be
seen to be irrelevant in the limit we will consider, we start with a Gaussian,
which is easy to handle analytically:
This packet has a mean momentum \({p_0} = \hbar {k_0}\), a mean position
○ (Step5, Shankar) Find the projection
♦ (My Commentary) Since \(U\left( {x,t\;;x'} \right)\) cannot be calculated, in equation \(\psi \left( {x,t} \right)\) is calculated first.
♦ The second integral vanishes (to an excellent approximation) since \({\psi _I}\left( x \right)\) is nonvanishing far to the left of \(x = 0\), while \(\theta \left( x \right)\) is nonvanishing only for \(x > 0\).
(My Commentary)
♦ the second piece of the first integral also vanishes since \({\psi _I}\) in \(k\) space is peaked around \(k = + {k_0}\) and is orthogonal to (left-going) negative momentum states.(I don’t understand ???. The contents of Golwala's Lecture Note are relatively easy to understand, and are cited in the box below.)
[We can ignore the \(\theta \left( { - x} \right)\) factor in Eq. (5.4.13) since it equals 1 where \({\psi _I}\left( x \right) \ne 0\).]
\(\theta \left( { - x} \right) = 1\) and \({\psi _I}\left( x \right) \ne 0\) for \(x < 0\)
Is just the Fourier transform of \({\psi _I}\)
[Golwala’s Lecture Note]
The two terms are calculating the Fourier transform at \(k\) of \({\psi _I}\left( {x,0} \right)\) for positive and negative \(k\), respectively. We know from our discussion of Gaussian wave packets for the free particle that the Fourier transform of our initial state is By shankar’s (1.10.31) and (1.10.32),
where we have made the simple change of variables to \(k = p/\hbar \) to match up with the way we index the states here with \(k\). Since \(\frac{{{\sigma _k}}}{{{k_0}}} = \frac{{{\sigma _p}}}{{{p_0}}} \ll 1\), it holds that the Gaussian essentially vanishes for \(k < 0\). (My Commentary : \({k_0} > 0\) and \({\sigma _k}\) is very small, so there is little effect of \(k < 0\) in gaussian distribution. Therefore, (My Commentary : I don't know exactly, but if \(A = 1/\sqrt {2\pi } \), it seems that \(\sqrt {2\pi } \) exists to make . \(A = 1/\sqrt {2\pi } \) is covered later.) |
|---|
○ (Step6, Shankar) Append to each coefficient a(E) a time dependence e^(- iEt/ℏ) and get ψ(x,t) at any future time.
Step 1 :
Step 2 :
Step 3 :
Step 4 :
You can convince yourself that if we set t
= 0 above we regain \({\psi
_I}\left( x \right)\), which corroborates our
choice \(A =
\frac{1}{{\sqrt {2\pi } }}\)
♦ Calculate \(A = \frac{1}{{\sqrt{2\pi } }}\) at \(t = 0\), \(\psi \left({x,t} \right) = {\psi _I}\left( {x,0} \right)\)
○ (Step7, Shankar) Identify \({\psi _R}\) and \({\psi _T}\) in \(\psi \left( {x,t \to \infty } \right)\) and determine \(R\) and \(T\) using Shankar’s Eqs. (5.4.3) and (5.4.4).
Consider the first of the three terms. If \(\theta \left( { - x} \right)\) were absent, we would be propagating the original Gaussian. After replacing \(x\) by \(x + a\) in Eq. (5.1.15), and inserting the\(\;\theta \left( { - x} \right)\) factor, the first term of \(\psi \left( {x,t} \right)\) is
Since the Gaussian \(G\left( { - a,{k_0},t} \right)\) is centered at \(x = - a + \frac{{\hbar {k_0}t}}{m} \simeq \frac{{\hbar {k_0}t}}{m}\) as \(t \to \infty \), and \(\theta \left( { - x} \right)\) vanishes for \(x > 0\),the product \(\theta G\) vanishes. Thus the initial packet has disappeared and in its place are the reflected and transmitted packets given by the next two terms. In the middle term if we replace \(B/A\), which is a function of \({k_1}\) , by its value \({\left( {B/A} \right)_0}\) at \({k_1} = {k_0}\) (because \(a\left( {{k_1}} \right)\) is very sharply peaked at \({k_1} = {k_0}\)) and pull it out of the integral, changing the dummy variable from \({k_1}\) to \( - {k_1}\) , it is easy to see that apart from the factor \({\left( {B/A} \right)_0}\theta \left( { - x} \right)\) up front, the middle term represents the free propagation of a normalized Gaussian packet that was originally peaked at \(x = + a\) and began drifting to the left with mean momentum \( - \hbar {k_0}\) . Thus
As \(t \to \infty \), we can set \(\theta \left( { - x} \right)\) equal to 1, since \(G\) is centered at \(x = a - \frac{{\hbar {k_0}t}}{m} \simeq - \frac{{\hbar {k_0}t}}{m}\). Since the Gaussian \(G\) has unit norm, we get from Eqs. (5.4.3) and (5.4.9),
This formula is exact only when the incident packet has a well-defined energy \({E_0}\), that is to say, when the width of the incident Gaussian tends to infinity. But it is an excellent approximation for any wave packet that is narrowly peaked in momentum space.
To find \(T\), we can try to evaluate the third piece. But there is no need to do so, since we know that
♦ Consider the unnormalized eigenstate
The incoming plane wave \({A_0}{e^{i{k_0}x}}\) has a probability current associated with it equal to
while the currents associated with the reflected and transmitted pieces are
(My commentary : shankar describes \({j_R} = \frac{{\hbar {k_0}}}{m}{\left| {{B_0}} \right|^2}\), and I calculate \({j_R} = - \frac{{\hbar {k_0}}}{m}{\left| {{B_0}} \right|^2}\). If the direction is neglected, the quantity is the same in both equations. )
the reflection and transmission probabilities (\(R\) and \(T\))
(My commentary : (\(R\) and \(T\)) are probabilities, so they should be positive real numbers. Therefore, the direction is ignored in eq. (5.4.26).)